Force-Distance Spectroscopy
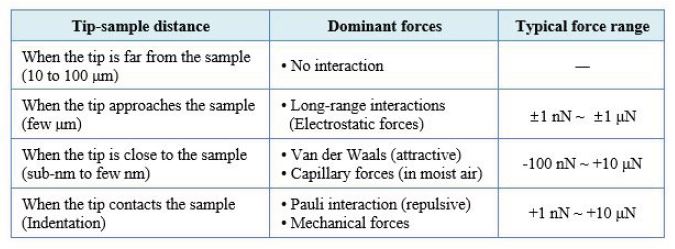
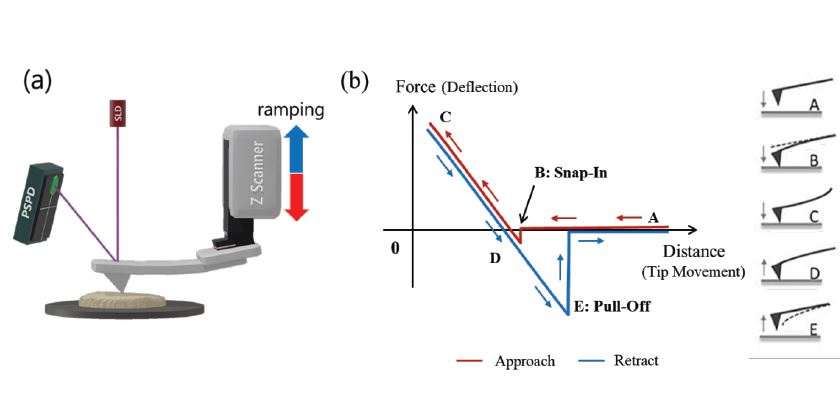
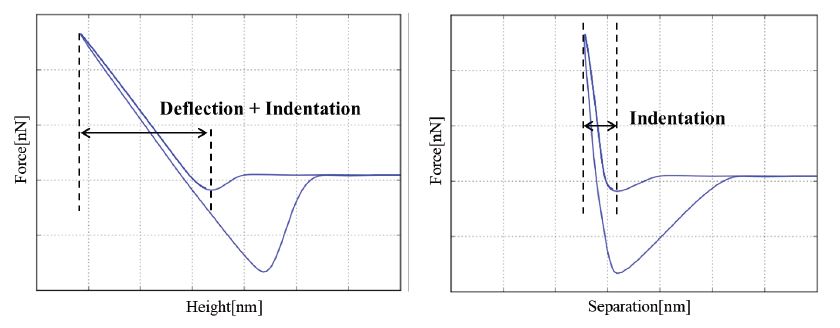
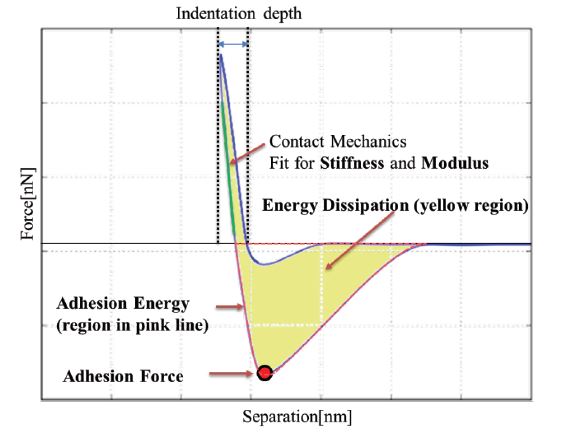
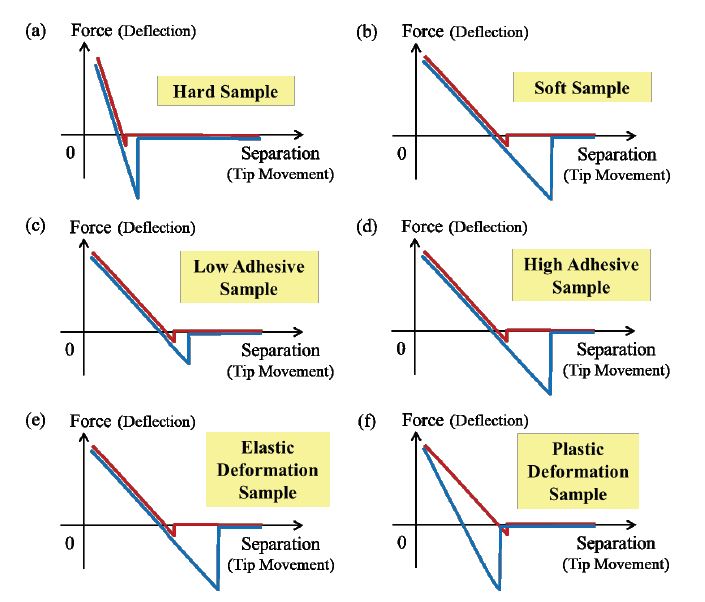
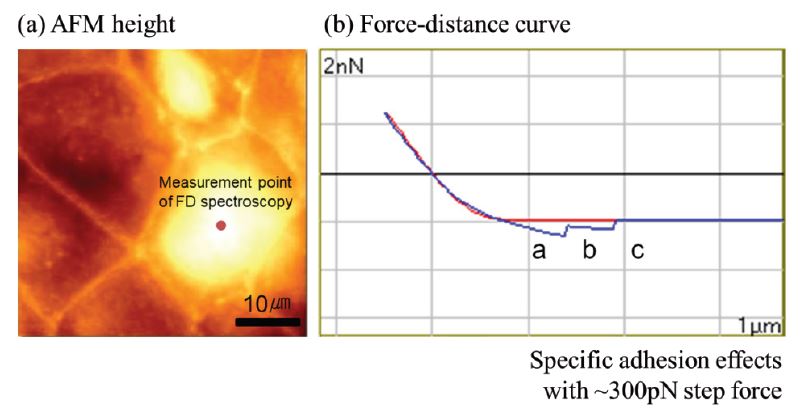
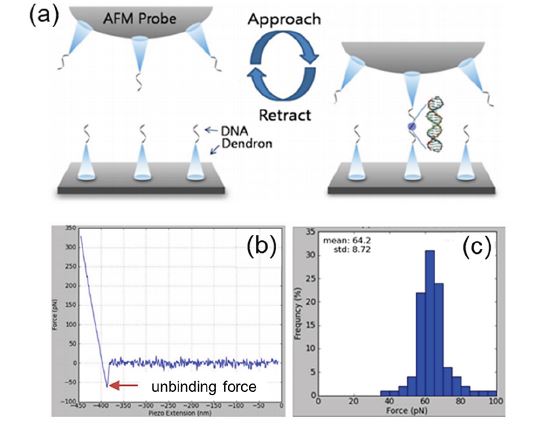
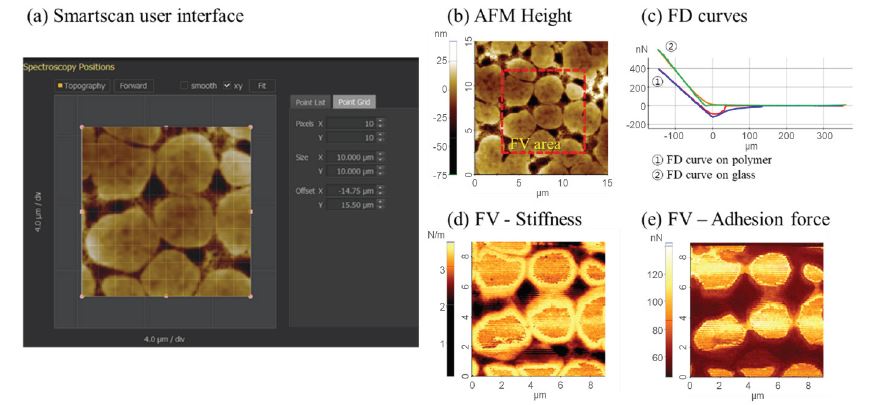
Here, Force distance (FD) spectroscopy is a straightforward and reliable technique to quantitatively study nanomechanical properties such as Young’s modulus and adhesion force on a variety of samples. Therefore, FD spectroscopy has become a fundamental characterization tool in several fields of research, including polymer science, biochemistry, and biology.